

An example of a uniform plane wave propagating in a lossless medium is shown in Figure \(\PageIndex<1>\). 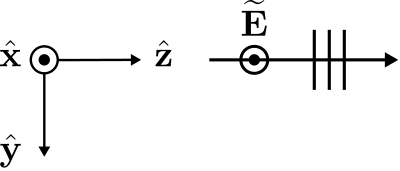 Figure \(\PageIndex<1>\): The plane wave described by Equation \ref. (CC BY-SA 4.0; C. Wang) This wave is expressed in the indicated coordinate system as follows: \[\widetilde = \hat E_0 e^ \label \] This is a phasor-domain expression for the electric field intensity, so \(E_0\) is a complex-valued number representing the magnitude and reference phase of the sinusoidally-varying wave. The term “reference phase” is defined as the phase of \(\widetilde\) at the origin of the coordinate system. Since the phase propagation constant \(\beta\) is real and positive, this wave is traveling in the \(+\hat\) direction through simple lossless media. Note that electric field intensity vector is linearly polarized in a direction parallel to \(\hat\). Depending on the position in space (and, for the physical time-domain waveform, time), \(\widetilde\) points either in the \(+\hat\) direction or the \(-\hat\) direction. Let us be a bit more specific about the direction of the vector \(\widetilde\). To do this, let us define the reference polarization to be the direction in which \(\widetilde\) points when \(\mbox\left\
Figure \(\PageIndex<1>\): The plane wave described by Equation \ref. (CC BY-SA 4.0; C. Wang) This wave is expressed in the indicated coordinate system as follows: \[\widetilde = \hat E_0 e^ \label \] This is a phasor-domain expression for the electric field intensity, so \(E_0\) is a complex-valued number representing the magnitude and reference phase of the sinusoidally-varying wave. The term “reference phase” is defined as the phase of \(\widetilde\) at the origin of the coordinate system. Since the phase propagation constant \(\beta\) is real and positive, this wave is traveling in the \(+\hat\) direction through simple lossless media. Note that electric field intensity vector is linearly polarized in a direction parallel to \(\hat\). Depending on the position in space (and, for the physical time-domain waveform, time), \(\widetilde\) points either in the \(+\hat\) direction or the \(-\hat\) direction. Let us be a bit more specific about the direction of the vector \(\widetilde\). To do this, let us define the reference polarization to be the direction in which \(\widetilde\) points when \(\mbox\left\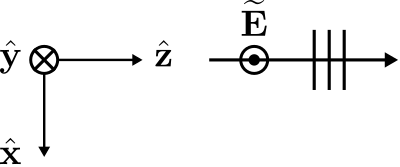 Figure \(\PageIndex\): The same plane wave described in a rotated coordinate system, yielding Equation \ref. (CC BY-SA 4.0; C. Wang) At first glance, it appears that the reference polarization has changed; however, this is due entirely to our choice of coordinate system. That is, the reference polarization is precisely the same; it is only the coordinate system used to describe the reference polarization that has changed. Let us now rotate the \(+z\) axis of the coordinate system around the \(x\) axis into the position originally occupied by the \(-z\) axis. Now the very same wave is expressed as \[\widetilde = +\hat E_0 e^ \label \] This is illustrated in Figure \(\PageIndex\).
Figure \(\PageIndex\): The same plane wave described in a rotated coordinate system, yielding Equation \ref. (CC BY-SA 4.0; C. Wang) At first glance, it appears that the reference polarization has changed; however, this is due entirely to our choice of coordinate system. That is, the reference polarization is precisely the same; it is only the coordinate system used to describe the reference polarization that has changed. Let us now rotate the \(+z\) axis of the coordinate system around the \(x\) axis into the position originally occupied by the \(-z\) axis. Now the very same wave is expressed as \[\widetilde = +\hat E_0 e^ \label \] This is illustrated in Figure \(\PageIndex\). 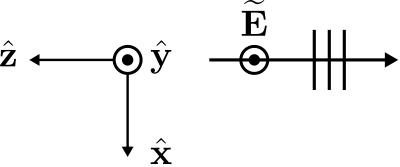 Figure \(\PageIndex\): The same plane wave described in yet another rotation of the coordinate system, yielding Equation \ref. (CC BY-SA 4.0; C. Wang) At first glance, it appears that the direction of propagation has reversed; but, again, it is only the coordinate system that has changed, and not the direction of propagation. Summarizing: Equations \ref, \ref, and \ref all represent the same wave. They only appear to be different due to our choice for the orientation of the coordinate system in each case. Now consider the same thought experiment for the infinite number of cases in which the wave does not propagate in one of the three basis directions of the Cartesian coordinate system. One situation in which we face this complication is when the wave is obliquely incident on a surface. In this case, it is impossible to select a single orientation of the coordinate system in which the directions of propagation, reference polarization, and surface normal can all be described in terms of one basis vector each. To be clear: There is no fundamental limitation imposed by this slipperiness of the coordinate system. However, practical problems such as the oblique-incidence scenario described above are much easier to analyze if we are able to express waves in a system of coordinates which always yields the same expressions. Fortunately, this is easily accomplished using ray-fixed coordinates. Ray-fixed coordinates are a unique set of coordinates that are determined from the characteristics of the wave, as opposed to being determined arbitrarily and separately from the characteristics of the wave. The ray-fixed representation of a uniform plane wave is: \[\boxed< \widetilde() = \hat E_0 e^\cdot> > \label \] This is illustrated in Figure \(\PageIndex\).
Figure \(\PageIndex\): The same plane wave described in yet another rotation of the coordinate system, yielding Equation \ref. (CC BY-SA 4.0; C. Wang) At first glance, it appears that the direction of propagation has reversed; but, again, it is only the coordinate system that has changed, and not the direction of propagation. Summarizing: Equations \ref, \ref, and \ref all represent the same wave. They only appear to be different due to our choice for the orientation of the coordinate system in each case. Now consider the same thought experiment for the infinite number of cases in which the wave does not propagate in one of the three basis directions of the Cartesian coordinate system. One situation in which we face this complication is when the wave is obliquely incident on a surface. In this case, it is impossible to select a single orientation of the coordinate system in which the directions of propagation, reference polarization, and surface normal can all be described in terms of one basis vector each. To be clear: There is no fundamental limitation imposed by this slipperiness of the coordinate system. However, practical problems such as the oblique-incidence scenario described above are much easier to analyze if we are able to express waves in a system of coordinates which always yields the same expressions. Fortunately, this is easily accomplished using ray-fixed coordinates. Ray-fixed coordinates are a unique set of coordinates that are determined from the characteristics of the wave, as opposed to being determined arbitrarily and separately from the characteristics of the wave. The ray-fixed representation of a uniform plane wave is: \[\boxed< \widetilde() = \hat E_0 e^\cdot> > \label \] This is illustrated in Figure \(\PageIndex\). 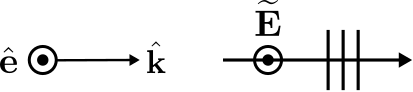 Figure \(\PageIndex\): A plane wave described in ray-fixed coordinates, yielding Equation \ref. (CC BY-SA 4.0; C. Wang) In this representation, \(\) is the position at which \(\widetilde\) is evaluated, \(\hat\) is the reference polarization expressed as a unit vector, and \(\) is the unit vector \(\hat\) in the direction of propagation, times \(\beta\); i.e.: \[ \triangleq \hat\beta \nonumber \] Consider Equation \ref as an example. In this case, \(\hat=\hat\), \( = \hat\beta\), and (as always) \[ = \hatx +\haty +\hatz \nonumber \] Thus, \(\cdot=\beta z\), as expected. In ray-fixed coordinates, a wave can be represented by one – and only one – expression, which is the same expression regardless of the orientation of the “global” coordinate system. Moreover, only two basis directions (namely, \(\hat\) and \(\hat\)) must be defined. Should a third coordinate be required, either \(\hat \times \hat\) or \(\hat \times \hat\) may be selected as the additional basis direction. Note that the first choice has the possibly-useful feature that is the reference polarization of the magnetic field intensity \(\widetilde\). A general procedure for recasting the ray-fixed representation into a “coordinate-bound” representation is as follows. First, we represent \(\) in the new fixed coordinate system; e.g.: \[ = k_x\hat + k_y\hat + k_z\hat \nonumber \] where \[\begin k_x &\triangleq \beta\hat\cdot\hat \\ k_y &\triangleq \beta\hat\cdot\hat \\ k_z &\triangleq \beta\hat\cdot\hat\end \nonumber \] Then, \[\cdot = k_x x + k_y y + k_z z \nonumber \] With this expression in hand, Equation \ref may be rewritten as: \[\widetilde = \hat E_0 e^ e^ e^ \nonumber \] If desired, one can similarly decompose \(\hat\) into its Cartesian components as follows: \[\hat = \left(\hat\cdot\hat\right)\hat + \left(\hat\cdot\hat\right)\hat + \left(\hat\cdot\hat\right)\hat \nonumber \] Thus, we see that the ray-fixed representation of Equation \ref accommodates all possible combinations of direction of propagation and reference polarization.
Figure \(\PageIndex\): A plane wave described in ray-fixed coordinates, yielding Equation \ref. (CC BY-SA 4.0; C. Wang) In this representation, \(\) is the position at which \(\widetilde\) is evaluated, \(\hat\) is the reference polarization expressed as a unit vector, and \(\) is the unit vector \(\hat\) in the direction of propagation, times \(\beta\); i.e.: \[ \triangleq \hat\beta \nonumber \] Consider Equation \ref as an example. In this case, \(\hat=\hat\), \( = \hat\beta\), and (as always) \[ = \hatx +\haty +\hatz \nonumber \] Thus, \(\cdot=\beta z\), as expected. In ray-fixed coordinates, a wave can be represented by one – and only one – expression, which is the same expression regardless of the orientation of the “global” coordinate system. Moreover, only two basis directions (namely, \(\hat\) and \(\hat\)) must be defined. Should a third coordinate be required, either \(\hat \times \hat\) or \(\hat \times \hat\) may be selected as the additional basis direction. Note that the first choice has the possibly-useful feature that is the reference polarization of the magnetic field intensity \(\widetilde\). A general procedure for recasting the ray-fixed representation into a “coordinate-bound” representation is as follows. First, we represent \(\) in the new fixed coordinate system; e.g.: \[ = k_x\hat + k_y\hat + k_z\hat \nonumber \] where \[\begin k_x &\triangleq \beta\hat\cdot\hat \\ k_y &\triangleq \beta\hat\cdot\hat \\ k_z &\triangleq \beta\hat\cdot\hat\end \nonumber \] Then, \[\cdot = k_x x + k_y y + k_z z \nonumber \] With this expression in hand, Equation \ref may be rewritten as: \[\widetilde = \hat E_0 e^ e^ e^ \nonumber \] If desired, one can similarly decompose \(\hat\) into its Cartesian components as follows: \[\hat = \left(\hat\cdot\hat\right)\hat + \left(\hat\cdot\hat\right)\hat + \left(\hat\cdot\hat\right)\hat \nonumber \] Thus, we see that the ray-fixed representation of Equation \ref accommodates all possible combinations of direction of propagation and reference polarization.
A uniform plane wave exhibiting a reference polarization of \(\hat\) propagates away from the \(z\)-axis. Develop representations of this wave in ray-fixed and global Cartesian coordinates.
As always, the ray-fixed representation is given by Equation \ref